◆ lw_dist2d_seg_arc()
| int lw_dist2d_seg_arc | ( | const POINT2D * | A1, |
| const POINT2D * | A2, | ||
| const POINT2D * | B1, | ||
| const POINT2D * | B2, | ||
| const POINT2D * | B3, | ||
| DISTPTS * | dl | ||
| ) |
Calculate the shortest distance between an arc and an edge.
Line/circle approach from http://stackoverflow.com/questions/1073336/circle-line-collision-detection
Definition at line 1363 of file measures.c.
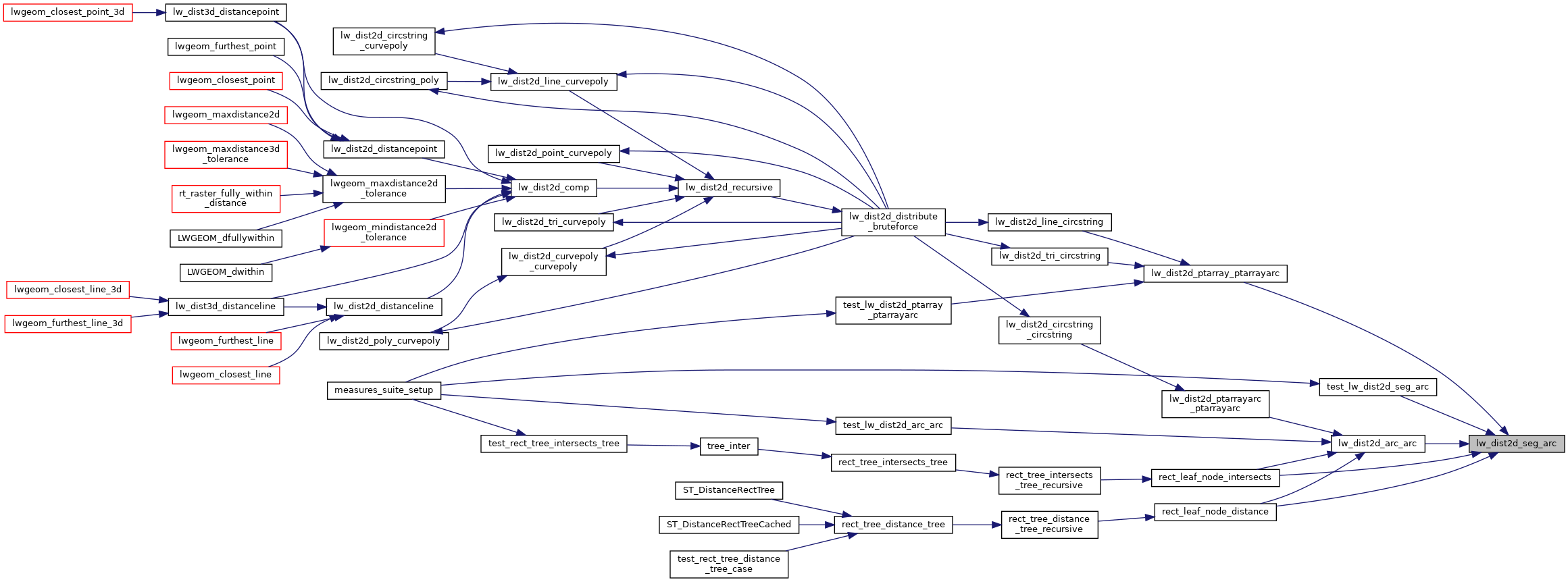
double lw_arc_center(const POINT2D *p1, const POINT2D *p2, const POINT2D *p3, POINT2D *result)
Determines the center of the circle defined by the three given points.
Definition: lwalgorithm.c:226
int lw_pt_in_seg(const POINT2D *P, const POINT2D *A1, const POINT2D *A2)
Returns true if P is between A1/A2.
Definition: lwalgorithm.c:93
int lw_arc_is_pt(const POINT2D *A1, const POINT2D *A2, const POINT2D *A3)
Returns true if arc A is actually a point (all vertices are the same) .
Definition: lwalgorithm.c:103
int lw_pt_in_arc(const POINT2D *P, const POINT2D *A1, const POINT2D *A2, const POINT2D *A3)
Returns true if P is on the same side of the plane partition defined by A1/A3 as A2 is.
Definition: lwalgorithm.c:83
int lw_dist2d_pt_arc(const POINT2D *P, const POINT2D *A1, const POINT2D *A2, const POINT2D *A3, DISTPTS *dl)
Definition: measures.c:1513
int lw_dist2d_pt_seg(const POINT2D *p, const POINT2D *A, const POINT2D *B, DISTPTS *dl)
lw_dist2d_comp from p to line A->B This one is now sending every occasion to lw_dist2d_pt_pt Before i...
Definition: measures.c:2306
int lw_dist2d_seg_seg(const POINT2D *A, const POINT2D *B, const POINT2D *C, const POINT2D *D, DISTPTS *dl)
Finds the shortest distance between two segments.
Definition: measures.c:1917
int lw_dist2d_pt_pt(const POINT2D *thep1, const POINT2D *thep2, DISTPTS *dl)
Compares incoming points and stores the points closest to each other or most far away from each other...
Definition: measures.c:2366
Definition: liblwgeom.h:389
References DIST_MIN, DISTPTS::distance, lw_arc_center(), lw_arc_is_pt(), lw_dist2d_distpts_init(), lw_dist2d_pt_arc(), lw_dist2d_pt_pt(), lw_dist2d_pt_seg(), lw_dist2d_seg_seg(), LW_FALSE, lw_pt_in_arc(), lw_pt_in_seg(), LW_TRUE, lwerror(), DISTPTS::mode, DISTPTS::p1, DISTPTS::p2, POINT2D::x, and POINT2D::y.
Referenced by lw_dist2d_arc_arc(), lw_dist2d_ptarray_ptarrayarc(), rect_leaf_node_distance(), rect_leaf_node_intersects(), and test_lw_dist2d_seg_arc().
Here is the call graph for this function:
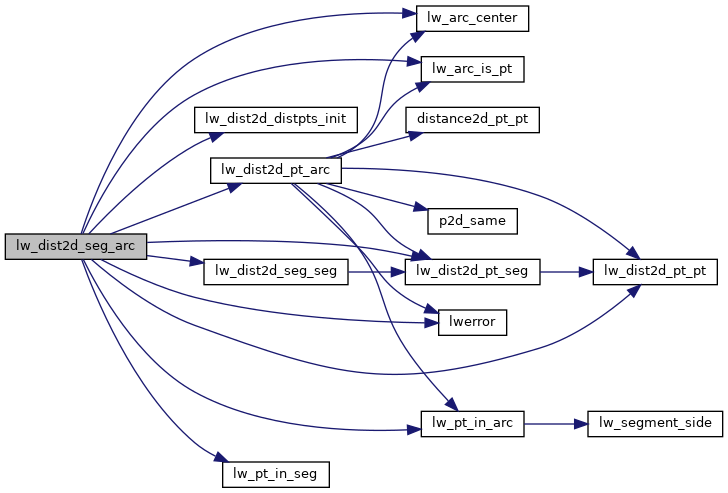
Here is the caller graph for this function: